
How Good Are Those Young-Earth Arguments?
A Close Look at Dr. Hovind's List of Young-Earth Arguments and Other Claims
by Dave E. Matson| Copyright © 1994-2002 |
![]()

| Copyright © 1994-2002 |
![]()
Previous |
Contents |
Next |
The following material has been taken from a sheet entitled Several Faulty Assumptions Are Used in all Radiometric Dating Methods. Carbon 14 is used for this example:, which was put out by Dr. Hovind.
Dr. Hovind (R1): The atmospheric C-14 is presently only 1/3 of the way to an equilibrium value which will be reached in 30,000 years. This nullifies the carbon-14 method as well as demonstrating that the earth is less than 10,000 years old.
R1. The above is offered as a simple fact of research. Knowing how faulty creationist "facts" can be, let's do a little research of our own. One suspects that the scientific world would not be using the carbon-14 method if it were so obviously flawed. Could it be that the whole scientific community has missed this point, or is it another case of creationist daydreaming?
This argument was popularized by Henry Morris (1974, p.164), who used some calculations done in 1968 by Melvin Cook to get the 10,000-year figure. In 1968 another creationist, Robert L. Whitelaw, using a greater ratio of carbon-14 production to decay, concluded that only 5000 years passed since carbon-14 started forming in the atmosphere!
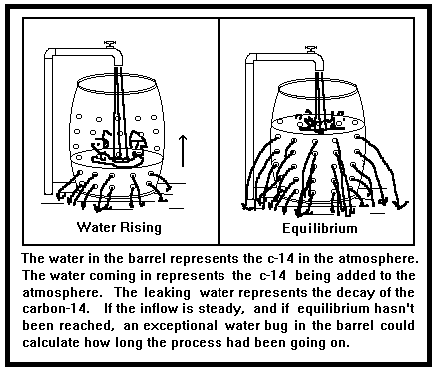
The argument may be compared to filling a barrel which has numerous small holes in its sides. We stick the garden hose in and turn it on full blast. The water coming out of the hose is analogous to the continuous production of carbon-14 atoms in the upper atmosphere. The barrel represents the earth's atmosphere in which the carbon-14 accumulates. The water leaking out the sides of the barrel represents the loss (mainly by radioactive decay) of the atmosphere's supply of carbon-14. Now, the fuller that barrel gets the more water is going to leak out the thoroughly perforated sides, just as more carbon-14 will decay if you have more of it around. Finally, when the water reaches a certain level in the barrel, the amount of water going into the barrel is equal to the amount leaking out the perforated sides. We say that the input and output of water is in equilibrium. The water level just sits there even though the hose is going full blast. (The barrel is made deep enough so that we don't have to worry about water overflowing the rim.)
Henry Morris argued that if we started filling up our empty barrel it would take 30,000 years to reach the equilibrium point. Thus, he concluded, if our Earth were older than 30,000 years the
incoming water should just equal the water leaking out. That is, the equilibrium point should have long since been reached given the present rate of carbon-14 production and the old age of the earth. The next step in Henry Morris' argument was to show that the water level in our barrel analogy was not in equilibrium, that considerably more water was coming in than leaking out. To that end, he quoted some authorities, including Richard Lingenfelter. Having accomplished that, Morris concluded that the barrel was still in the process of being filled up and that, given the present rate of water coming in and leaking out, the filling process began only 10,000 years ago.
It's a great argument except for one, little thing. The water is not coming out of the hose at a steady rate as our model assumed! Sometimes it slows down to a trickle so that much more water is leaking out the barrel than is coming in; sometimes it goes full blast so that a lot more water is coming into the barrel than is leaking out. Thus, the mere fact that the present rate of water coming in exceeds that of the water leaking out cannot be extrapolated back to a starting time. And, that destroys the entire argument. (See Figure 1).
|
Lingenfelter's paper was written in 1963, before the cycles of C-14 variation we described had been fully documented. The point is that fluctuations in the rate of C-14 production mean that at times the production rate will exceed the decay rate, while at other times the decay rate will be the larger.
(Strahler, 1987, p.158)
Lingenfelter actually attributed the discrepancy between the production and decay rates to possible variations in the earth's magnetic field, a conclusion which would have ruined Morris's argument. Henry Morris chose not to mention that portion of the paper! Creationists don't want their readers to be distracted with problems like that -- unless the cat is already out of the bag and something has to be said.
Tree-ring dating (see Topic 27) gives us a wonderful check on the radiocarbon dating method for the last 8000 years. That is, we can use carbon-14 dating on a given tree-ring (the 8000-year sequence having been assembled from the overlapping tree-ring patterns of living and dead trees) and compare the resulting age with the tree-ring date. A study of the deviations from the accurate tree-ring dating sequence shows that the earth's magnetic field has an important effect on carbon-14 production. When the dipole moment is strong, carbon-14 production is suppressed below normal; when it is weak, carbon-14 production is boosted above normal. What the magnetic field does is to partially shield the earth from cosmic rays which produce carbon-14 high in the atmosphere.
Contrary to creationist Barnes' totally discredited claims, which I've covered in Topic 11, the earth's magnetic field (dipole moment) has, indeed, increased and decreased over time. Strahler presents a graph of the earth's dipole moment going back 9000 years.
Figure 19.5, curve C, shows the dipole field strength calculated from measurements of magnetism of lava flows and of artifacts such as pottery and bricks, whose age can be determined. The curve is roughly fitted to mean values determined about every 500 to 1,000 years... The curve is roughly 180 degrees out of phase with the C-14 curve.
(Strahler, 1987, p.156)
The idea [that the fluctuating magnetic field affects influx of cosmic rays, which in turn affects C-14 formation rates] has been taken up by the Czech geophysicist, V. Bucha, who has been able to determine, using samples of baked clay from archeological sites, what the intensity of the earth's magnetic field was at the time in question. Even before the tree-ring calibration data were available to them, he and the archeologist, Evzen Neustupny, were able to suggest how much this would affect the radiocarbon dates. (Renfrew, p.76)
(Weber, 1982, p.27)
Thus, at least within the last 9000 years, the earth's magnetic field has fluctuated and those fluctuations have induced fluctuations in the production of carbon-14 to a noticeable extent. Therefore, as already noted, Dr. Hovind's claim that carbon-14 has been slowly building up towards a 30,000 year equilibrium is worthless. You now have the technical reason for the failure of Morris' model.
It may interest the reader to know that within this 9000-year period, where the radiocarbon method can be checked by tree-ring data, objects older than 400 BC receive a carbon-14 date which makes them appear younger than they really are! An uncorrected carbon-14 date of 6000 years for an object would actually mean that the object was 6700 years old. Seven hundred years or so is about as far as the carbon-14 method strays from tree-ring dating on the average. Individual dates given on a 1973 correlation chart (Bailey, 1989, p.100) show that objects with true ages between 4200 BC and 5400 BC would receive a carbon-14 date making them appear 500-900 years too young. As it turns out, we have a check on the carbon-14 production which goes back even further than 8000 years:
Evidence of past history of C-14 concentration in the atmosphere is now available through the past 22,000 years, using ages of lake sediments in which organic carbon compounds are preserved. Reporting before a 1976 conference on past climates, Professor Minze Stuiver of the University of Washington found that magnetic ages of the lake sediments remained within 500 years of the radiocarbon ages throughout the entire period. He reported that the concentration of C-14 in the atmosphere during that long interval did not vary by more than 10 percent (Stuiver, 1976, p. 835).
Thus, the available evidence is sufficient to validate the radiocarbon method of age determination with an error of about 10 percent for twice as long a period as the creation scenario calls for.
(Strahler, 1987, p.157)
Yes, the atmospheric content of carbon-14 can vary somewhat. The dipole moment of the earth's magnetic field, sunspot activity, the Suess effect, possible nearby supernova explosions, and even ocean absorption can have some effect on the carbon-14 concentration. However, these factors don't affect the radiocarbon dates by more than about 10-15 percent, judging from the above studies. Of course, when we reach the upper limit of the method, around 40,000 years for the standard techniques, we should allow for much greater uncertainty as the small amounts of C-14 remaining are much harder to measure.
Tree-ring data gives us a precise correction table for carbon-14 dates as far back as 8,000-9,000 years. The above study by Stuiver shows that the C-14 fluctuations in the atmosphere were quite reasonable as far back as 22,000 years ago. The earth's magnetic field seems to have the greatest effect on C-14 production, and there is no reason to believe that its strength was greatly different even 40,000 years ago. (For a refutation of Barnes' argument see Topic 11.)
Therefore, atmospheric variation in C-14 production is not a serious problem for the carbon-14 method. The evidence refutes Dr. Hovind's claim that the C-14 content of our atmosphere is in the middle of a 30,000-year buildup. Thus, we can dismiss this young-earth argument.
Dr. Hovind (R2): The C-14 decay rate is not constant. Several factors, including the 11-year sunspot cycle, affects its rate of decay.
R2. It is painfully obvious that Dr. Hovind knows next to nothing about carbon-14 dating! Changes in the sunspot cycle do have a noticeable, short-term effect on the rate of C-14 production inasmuch as sunspots are associated with solar flares, which produce magnetic storms on Earth, and the condition of the earth's magnetic field does affect the number of cosmic rays reaching the earth's upper atmosphere. (Carbon-14 is produced by energetic collisions between cosmic rays and molecules of nitrogen in the upper atmosphere.) Sunspots have absolutely nothing to do with the rate of C-14 decay, which defines the half-life of that radioactive element. Dr. Hovind has confused two completely different concepts.
Quantum mechanics, that stout pillar of modern physics, which has been verified in so many different ways that I couldn't begin to list them all even if I had them at hand, gives us no theoretical reason for believing that the C-14 rate of decay has changed or can be significantly affected by any reasonable process. We also have direct observation:
That radiocarbon ages agree so closely with tree-ring counts over at least 8000 years, when the observed magnetic effect upon the production rate of C-14 is taken into account, suggests that the decay constant itself can be assumed to be reliable.
(Strahler, 1987, p.157)
Since 8000 years is almost two half-lives for carbon-14, it's half-life being 5730 years (plus or minus 40 years), we have excellent observational evidence that the decay rate is constant. We also have laboratory studies which support the constancy of all the decay rates used in radiometric dating.
A great many experiments have been done in attempts to change radioactive decay rates, but these experiments have invariably failed to produce any significant changes. It has been found, for example, that decay constants are the same at a temperature of 2000 degrees C or at a temperature of -186 degrees C and are the same in a vacuum or under a pressure of several thousand atmospheres. Measurements of decay rates under differing gravitational and magnetic fields also have yielded negative results. Although changes in alpha and beta decay rates are theoretically possible, theory also predicts that such changes would be very small [Emery, 1972] and thus would not affect dating methods. Under certain environmental conditions, the decay characteristics of C-14, Co-60, and Ce-137, all of which decay by beta emission, do deviate slightly from the ideal random distribution predicted by current theory [Anderson, 1972; Anderson & Spangler, 1973], but changes in the decay constants have not been detected.
There is a fourth type of decay that can be affected by physical and chemical conditions, though only very slightly. This type of decay is electron capture (e.c. or K-capture), in which an orbital electron is captured by the nucleus and a proton is converted into a neutron. Because this type of decay involves a particle outside the nucleus, the decay rate may be affected by variations in the electron density near the nucleus of the atom. For example, the decay constant of Be-7 in different beryllium chemical compounds varies by as much as 0.18 percent [Emery, 1972, 64]. The only isotope of geologic interest that undergoes e.c. decay is K-40, which is the parent isotope in the K-Ar method. Measurements of the decay rate of K-40 in different substances under various conditions indicate that variations in the chemical and physical environment have no detectable effect on its e.c. decay constant.
(Dalrymple, 1984, p.88)
Believe it or not, a number of creationist attacks against radiometric decay rates are aimed at a kind of "decay" called internal conversion (IC), which has absolutely nothing to do with the radiometric dating methods (Dalrymple, 1984, p.88). Harold Slusher, a prominent member of the Institute for Creation Research, claimed that "Experiments have shown that the decay rates of cesium 133 and iron 57 vary, hence there may be similar variations in other radioactive decay rates." (Slusher, 1981, p.22, 49; from Brush)
These are both stable isotopes so there is no decay rate to be changed. This statement merely reveals Slusher's ignorance of nuclear physics. (Gamma decay of an excited state of iron 57 has been studied, but this has nothing to do with the kinds of decays used in radiometric dating.)
(Brush, 1982, p.52)
DeYoung [1976] lists 20 isotopes whose decay rates have been changed by environmental conditions, alluding to the possible significance of these changes to geochronology, but the only significant changes are for isotopes that "decay" by internal conversion. These changes are irrelevant to radiometric dating methods.
(Dalrymple, 1984, p.88)
Keep an eye on those creationists! They will switch tracks faster than you can say "tiddlywinks." One moment they're talking about the radioactive decay of the nuclides involved in geochronology, and, in the next moment, they're passing out examples of IC decay in stable isotopes. Morris (1974) claimed that free neutrons might change the decay rates. However, Henry Morris, that icon of creationism, only demonstrated that he knew no more about radiometric dating than does Dr. Hovind today. "...[Morris'] arguments show that he does not understand either neutron reactions or radioactive decay." (Dalrymple, 1984, pp.88-89). Free neutrons might change one element into another, but the decay rates all remain true to their elements.
Another attempt by Morris invokes neutrinos.
Morris [1974] also suggests that neutrinos might change decay rates, citing a column by Jueneman (72) in Industrial Research. The subtitle of Jueneman's columns, which appear regularly, is, appropriately, "Scientific Speculation." He speculates that neutrinos released in a supernova explosion might have "reset" all the radiometric clocks. Jueneman describes a highly speculative hypothesis that would account for radioactive decay by interaction with neutrinos rather than by spontaneous decay, and he notes that an event that temporarily increased the neutrino flux might "reset" the clocks. Jueneman, however, does not propose that decay rates would be changed, nor does he state how the clocks would be reset; in addition, there is no evidence to support his speculation.
(Dalrymple, 1984, p.89)
There was also an attempt by Slusher and Rybka to invoke neutrinos. Those mysterious neutrinos seem to be a hot topic!
Slusher (117) and Rybka (110) also propose that neutrinos can change decay rates, citing an hypothesis by Dudley (40) that decay is triggered by neutrinos in a "neutrino sea" and that changes in the neutrino flux might affect decay rates. This argument has been refuted by Brush (20), who points out that Dudley's hypothesis not only requires rejection of both relativity and quantum mechanics, two of the most spectacularly successful theories in modern science, but is disproved by recent experiments. Dudley himself rejects the conclusions drawn from his hypothesis by Slusher (117) and Rybka (110), noting that the observed changes in decay rates are insufficient to change the age of the Earth by more than a few percent (Dudley, personal communication, 1981, quoted in 20, p.51). Thus, even if Slusher and Rybka were correct--which they are not--the measured age of the Earth would still exceed 4 billion years.
(Dalrymple, 1984, p.89)
Dalrymple goes on to debunk several other creationists attacks on the reliability of the radiometric decay rates used in geochronology. Judging from the above, it is easy to see that creationists are indulging in wild fishing expeditions. Compare their flighty arguments to the solid support provided by theoretical work, laboratory testing, and, for the shorter half-lives, actual observation, and add to that the statistical consistency of the dates obtained, including numerous cross-checks between different "clocks," and only one conclusion is left. The radiometric decay rates used in dating are totally reliable. They are one of the safest bets in all of science.
Dr. Hovind (R3): The initial C-14 content cannot be known. Different parts of the same sample often yield different ratios of C-14/C-12. Various living samples give very different ratios.
R3. With at least one notable exception on the books, plants and animals get their carbon-14 from the atmosphere. Plants take it in directly, and animals eat the plants. Thus, it gets passed up the food chain. It is not surprising, therefore, to find that the carbon-14 in living plants and animals is in reasonable equilibrium with the atmospheric carbon-14. Some creationists, however, have claimed that certain plants can reject carbon-14 in favor of carbon-12. Because of the chemical similarity of carbon-14 and carbon-12, it is unlikely that such plants could deviate much from the ratio of C-14 to C-12 found in the atmosphere. Neither freak cases nor small deviations pose much of a problem for radiocarbon dating, which, after all, works well with a wide variety of plant and animal species. Hence, we only have to worry about the initial concentration of C-14 in the atmosphere. Topic R1 shows that the level of C-14 in the atmosphere has not varied appreciably over tens of thousands of years. Therefore, the initial C-14 content is known for any reasonable sample!
The notable exception involves certain mollusks, which get much of their carbon from dissolved limestone. Since limestone is very old it contains very little carbon-14. Thus, in getting some of their carbon from limestone, these mollusks "inherit" some of the limestone's old age! That is, the limestone carbon skews the normal ratio between C-12 and C-14 found in living things. No problem! If one dates such mollusks, one must be extra careful in interpreting the data. Not every mollusk shell presents such problems, and the dating of other material might yield a cross-check. Further study might even allow correction tables. The discovery has strengthened the carbon-14 method, not weakened it! By the way, shouldn't the creationist be worried over the old, carbon-14 age of the limestone? Why is it that limestone has so little C-14 in it?
Different parts of the same sample may, indeed, yield different C-14/C-12 ratios. Partial contamination, say of a block of wood, may affect its different parts to different degrees. Insect burrows, cracks, and partial decay may allow contamination later on to affect those portions of the sample unequally. However, there are laboratory techniques, often ingenious, for dealing with such problems. If the sample shows evidence of being hopelessly contaminated it is pitched.
Some samples, such as a section of a tree trunk, may well contain material of considerably different ages. The interior portion of a tree trunk could easily be several hundred years older than the outer portions. Once again, the C-14/C-12 ratios would reflect this difference in age.
In summing up this point, we do know within good limits what the initial C-14 was for any reasonable sample. A sample will not have different ratios of carbon unless it has been contaminated or reflects a genuine range of ages.
Dr. Hovind (R4): It is very difficult or impossible to prove that a given sample has not been contaminated. Parent or daughter products could have leached in or out of the sample.
R4. In the case of carbon-14 dating, the daughter product is ordinary nitrogen and plays no role in the dating process. We are only interested in tallying the original C-14 still present in the sample, the surviving "parent" isotope. The C-14 that is incorporated in the carbon structure of cellulose and the other structural materials of living plants and animals is not going to do much migrating after burial. If structural carbon migrated easily there soon wouldn't be any cellulose, lignin, chitin (or other structural carbon compounds) left in the soil! A piece of wood, for example, would soon turn into a formless cloud of graphite or soot in the soil, with perhaps a little ash marking the original shape! Clearly, that is not something which normally happens. Residues or solutions which do migrate can usually be washed out of the structural matrix of the sample with various chemicals.
To put it another way, we might imagine a piece of buried wood as being something like a sponge. Any carbon-containing liquid originally possessed by that sponge might well leak over time and be replaced by something else. However, unless the sponge itself disintegrates, the carbon which holds its fibers together must stay put. Thus, by choosing a sample that is structurally intact, one may rule out any significant loss of C-14. If the liquid impurities in our sponge can be washed and squeezed out, or estimated in some way, then we may be able to date the sponge (structural component of our sample) itself and get a good date even if non-structural carbon-14 had been lost in a manner that would upset the isotope ratio.
A sample, of course, can be contaminated if organic material rich in fresh atmospheric C-14 soaks or diffuses into it. Such contamination may occur in the ground or during the processing of the sample in the laboratory. However, such contamination will make the sample appear younger than its true age. Consequently, with regards to carbon-14 dating, creationists are barking up the wrong tree on the contamination issue!
Laboratories, of course, do have techniques for identifying and correcting contamination. There are various methods of cleaning the material, and the activity of each rinse can be measured. Lab contamination and technique can be checked by running blanks. A careful choice of samples will often minimize contamination. Dating various portions of a sample is another kind of check that may be performed.
Often there are cross-checks. Samples from top to bottom of a peat bog gave reasonable time intervals (Science, vol.200, p.11). The calibrated C-14 method confirmed Egyptian records, and most of the Aegean dates which were cross-dated with Egyptian dates were confirmed (American Scientist, May-June 1982). The marvelous agreement with tree-ring data, after correction for variations in the earth's magnetic field, has already been mentioned.
Carbon-14 dating thus presents a deadly challenge to young-earth creationists. If an old date is reasonably accurate, they're out of business; if an old date is bad due to contamination, then they are still out of business because the true date is most likely older still. It hardly seems fair, but that's the way it is. With that in mind, let's look at a few carbon-14 dates.
Egyptian barley samples have been found which date to 17,000-18,300 years old (Science, April 7, 1978). On page 1346 the author explains some of the professional care which stands behind his use of the carbon-14 method.
A wooden walkway buried in a peat bog in England has been dated to about 4000 BC by the carbon-14 method (Scientific American, August 1990, p.30). Odd, that Noah's flood neither destroyed it nor deposited thick sediments on top of it! Jennifer Hillam of the University of Sheffield and Mike Baillie of Queen's University of Belfast and their colleagues were able to date the walkway by a second method, i.e., tree-ring dating. They found out that the walkway, known as the Sweet Track, was built from trees felled in the winter of 3807-3806 BC. Pretty close agreement, huh?
Stonehenge, as dated by carbon-14, was built over a period from 1900 BC to 1500 BC -- long before the Druids came to England. Astronomer Gerald Hawkins found, after careful computer calculations, that the arrangement of the stones at Stonehenge are aligned with key positions of the sun and moon as they were almost 4000 years ago. (Weber, 1982, p.29). Thus, we have another remarkable confirmation of the C-14 method.
When did the volcano that destroyed Thera (and probably the Minoan culture as well) explode? Radiocarbon dating of seeds and wood buried in the ash, done by scientists at the University of Pennsylvania, pointed to no later than 1600 BC. Being that this was one of the biggest volcanic eruptions in recorded history, it almost certainly caused worldwide cooling which would, in turn, affect tree growth. Sure enough, the growth rings among oaks buried in Ireland's bogs show the effect of unusual cooling from 1628-1618 BC. Nor was that just an effect of local weather conditions. The bristlecone pines in the White Mountains of California show the same thing. A third estimate came from studies in Greenland. "In 1987 Danish geologists examining signs of volcanic acidity in the Greenland ice sheet concluded that the Thera volcano erupted in 1645 B.C., give or take 20 years." (Biblical Archaeology Review, Jan/Feb 1991, p.48). Thus, we have a remarkable agreement between three different methods, all within two or three percentage points of each other!
Trees buried by the last advance of glacial ice at Two Creeks, Wisconsin were dated at 11,850 years. (Strahler, 1987, p.251). Between those trees, which are buried in Valders red till, and an earlier, deeper layer of till, the Woodfordian gray till, lay the remains of a forest bed! What is a forest, including developed soil and rooted stumps, doing between two advances of ice? That could be an interesting question for someone who believes in only one "ice age." In 1878 Baron Gerard de Geer, a Swedish geologist, made a careful study of the annual varves left in European glacial lakes. By careful counting and cross-checking he was able to determine that the oldest glacial lakes, which would have formed at the start of the retreat of the ice, were 12,000 years old. Thus, we have a rough check between varves in glacial lakes and radiocarbon dating.
Richard Foster Flint, a professor of geology at Yale University and an expert on the Pleistocene epoch, was among the first to apply radiocarbon dating to glacial events. Collecting wood, bones and other organic material that had been covered over by the Laurentide Ice Sheet as it plowed across eastern and central North America, Flint collaborated with geophysicist Myer Rubin to demonstrate in 1955 that in most places the ice sheet achieved its greatest advance about 18,000 years ago, began to withdraw shortly thereafter and then hastened its retreat about 10,000 years ago.
(Chorlton, 1984, p.120)
Ancient cave art, at cueva de los caballos, near Castellon, Spain has been dated at about 6000 BC (The Times Atlas of World History [1978]).
On the wall of Gargas Cave in the French Pyrenees are the outlined hands of Ice Age artists which date to at least 12,000 years. Magnificent prehistoric cave art, comparable to that of the world-famous caves of Altamira, Spain and Lascaux, France, was recently discovered in southern France, in the Ardeche River canyon area (Los Angeles Times; Pasadena Star-News January 19, 1995). Its 300 paintings of such animals as bison, reindeer, rhinoceros, woolly rhinoceros, a panther, an owl, a hyena, bears, lions, horses, wild oxen, mammoths, wild goats and other animals is estimated to be between 19,000-22,000 years old. Sorry, no dinosaur drawings were reported! In Europe, cave art was at its height around 20,000 years ago. Some examples probably go back 30,000 years!
Dr. Hovind (R5): The C-14 cannot be accurately measured. It makes up less than one part per million in the atmosphere, and claiming to be able to measure accurately to 7 decimal places is not reasonable.
R5. This is similar to an argument put out by Harold Slusher (1981, p.45). Dr. Hovind adds the bizarre claim that something can't be measured accurately to seven decimal places. Such nonsense is answered by Dr. Dalrymple, an expert in radiometric dating, who noted that: "Modern counting instruments, available for more than two decades, are capable of counting the C-14 activity in a sample as old as 35,000 years in an ordinary laboratory, and as old as 50,000 years in laboratories constructed with special shielding against cosmic radiation. New techniques using accelerators and highly sensitive mass spectrometers, now in the experimental stage, have pushed these limits back to 70,000 or 80,000 years..." (Dalrymple, 1984, pp.86-87).
We can also explore this issue from first principles.
Given that the half-life of carbon-14 is 5730 years, one can calculate that 4 billion C-14 atoms will produce 1 decay per minute on the average. Converting the 4 billion atoms to grams (a nickel weighs 5 grams), we get 0.000000000000093 grams of carbon-14. Consequently, by tallying one click per minute on the Geiger counter, we can measure a whole lot further than 7 decimal places!
A 1-gram, fresh sample of carbon, containing the atmospheric concentration of one ten-billionth percent of carbon-14, will yield about 12 decays per minute. That figure follows directly from the mathematics and, as the atmospheric portion of carbon-14 given above is an approximation, is close enough to Dr. Hovind's present-day figure of 16 counts per minute per gram. Because of atomic bomb tests, the rate is slightly higher today, but the present rate would not apply to animals and plants which died before such tests. One book used a figure of about 13.5 decays per minute per gram for the pre-bomb rate. Consequently, a 64-gram sample of fresh carbon will still give about 7 clicks per minute after 40,000 years. Because of background radiation, that's about as far as one can normally go with this counting method. As noted above, Dr. Dalrymple would extend that to 50,000 years in special laboratories.
Once again, Dr. Hovind has relied on bad data. If you get your information from a creationist source, you'd better triple-check it! Errors get handed down in the creationist literature like the family jewels!
Dr. Hovind (R6): The shape of the curve of the line is based on too few real measurements to be reliable.
R6. It's not clear to me what Dr. Hovind is talking about. If he is referring to the carbon-14 decay curve then he has demonstrated, once again, his ignorance of radiometric dating.
The decay curve is mathematically determined by the fact that every atom of carbon-14 in a sample has the same chance of decaying during each second of time. That much is predicted by quantum mechanics, which is possibly the greatest of our modern, scientific revolutions.
The random character of radioactive decay is a special case of the indeterminacy of quantum theory, as was pointed out in 1928 by George Gamow, Ronald Gurney and Edward Condon. They showed that a particle held inside the nucleus by a "potential barrier" may be able to "tunnel through" the barrier and emerge on the other side, since if the barrier is finite the wave function of the particle is not completely localized and there is a finite probability that the particle will be outside the nucleus.
(Brush, 1982, p.42)
Since we are dealing with millions of C-14 atoms in even the smallest samples, the amount of C-14 remaining with respect to time will be an excellent approximation of an exponential decay curve. Statistics assure us of that. Indeed, it would be absurd to speak of the half-life of a radioactive isotope if it did not have a good exponential decay curve!
Once we have a good approximation of the half-life for carbon-14, its decay curve can be constructed with complete confidence. We don't need Egyptian mummies or what have you at that point. At that point it's just a routine exercise in math. If you want additional assurance that we have the correct half-life, then look at the close correlation between C-14 dates and tree-ring dates (after correcting for variances in C-14 production caused by changes in the earth's magnetic field). The snug fit indicates that the half-life of C-14 is stable and accurately known. Therefore, so is its decay curve.
Today, the half-lives of those radioactive elements used in dating are known to a few percent by careful laboratory study. So, there's no problem in getting an accurate decay curve.
Previous |
Contents |
Next |

| Home Page | Browse | Search | Feedback | Links |
| The FAQ | Must-Read Files | Index | Creationism | Evolution | Age of the Earth | Flood Geology | Catastrophism | Debates |